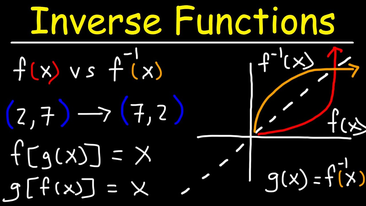
Inverse functions are intensively used in Mathematics, you can say there is always an inverse function of every function in Mathematics. Inverse function calculator by calculator-online.net can be best to find the inverse of a function. Whatever the function like Logritritum, then there is AntiLogarithm, there is Inverse of derivative is antiderivative, which is also known as the integration. There is almost every question in calculus, you need to do integration, its usage is extensive in the calculus.
The inverse calculator can be a great help to students to find the integral of any function. The same goes in Trigonometry, there is always an inverse function of ever trigonometric ratios like Sin, Cos, Tan, the Inverse trigonometric ratios are Sec, Cosec, and Cot. These Inverse trigonometric ratios are widely used in Mathematics. The inverse of a function calculator can be one of the best tools to find an inverse of function, this can be greatly helpful in solving many difficult questions.
In this article, we are discussing the practical implementation of the the Inverse function:
The Real Life Example of Inverse Function:
There are many real time examples of inverse functions in our real life, we are representing some of the examples, in this article, here we describe the implementation of the inverse function. Inverse function calculator can be greatly helpful in finding the inverse of a function:
- The inverse function calculator can be used in the proportionality question, for example if certain number of workers complete a work, and inversely when the number of workers is lesser than the more time would be take and if more worker are installed in the same work, then the less time would be spend in the same work.
- The same applies to the speed of a moving vessel like train, or a vehicle and the time taken to cover a certain distance. Inversely higher the speed the lesser time would be taken to complete the distance. When you find the value of a function, then you can use the inverse function calculator to find the inverse of the function.
A Real-time example:
Now consider a real-time example, it takes 8 days for 35 laborers to harvest a rice field plantation. How long will 20 laborers take to harvest the rice field plantation:
Solution of the above question:
- The 35 laborers take 8 days to harvest the rice field
- Now by the unitary method, the time is taken by the one worker = (35 × 8)days
- Now the time taken by the 20 workers would be = (35 × 8)/20
= 14 days required to completely harvest the rice field plantation
Now we can use the inverse function, if we want to reduce the number of days, then how many more workers do we need to completely harvest the rice field plantation. You can also solve the question to find the inverse of a function calculator, this can be great in solving your problem.
The second real-time example:
9 taps can fill a tank in 4 hours, so how long does it world take for 12 taps to fill the same tank at the same flow rate
The solution of the example:
- Now make the ratios of we get:
- x1/x2= y1/ y2
- 9/x = 12/4
- x= 3
- So the 12 tapes would only take 3 hours to fill the same tank to fill at the same rate of flow.
- We can use the inverse of this question, if we want to fill the tank in 2 hours at the same flow rate as how many tapes we need, we can also use the inverse function calculator to solve the same questions.
The different usage of the inverse function:
There are different usages of the inverse function calculator in real-time, and normally the inverse function calculator is used to cancel the effect of one function. You can also say to undo the effect of the other function. You can find the inverse of a function calculator easily by searching out, there are many types of inverse function available. You can say the subtraction is an inverse function to the addition and the same goes for the multiplication which is actually the inverse of the division.
You can see all these functions can cancel the effect of each other but we don’t realize this phenomenon of Mathematics, and we consider these functions separate entities and have no relation with each other. The same goes for the Celcius and the Fahrenheit scale, you can realize these functions are canceling each other’s effect but are used separately from each other.